This content originally appeared on HackerNoon and was authored by Phenomenology
:::info Author:
(1) Ahmed Farag Ali, Essex County College and Department of Physics, Faculty of Science, Benha University.
:::
Table of Links
Space-time quanta and Becken Universal bound
Space-time quanta and Spectral mass gap
Conclusion, Acknowledgments, and References
II. SPACE-TIME QUANTA AND BEKENSETIN UNIVERSAL BOUND
In this section, we investigate the physical properties of space-time quanta implied by Snyder’s algebra. It is clear that Eq. (1) only vanishes if there is no fundamental minimal/quantum length (i.e κℓP l = 0). This means non-commutative geometry would vanish if there is no minimal/quantum length. On the contrary, we find that the GUP commutation relation in Eq. (2) vanishes. The time-energy commutation relation of Eq. (2) vanishes when:
\

\ where E = p0 and Eκ represents the maximum bound on energy. The position-momentum commutation relation Eq. (2) vanishes when:
\
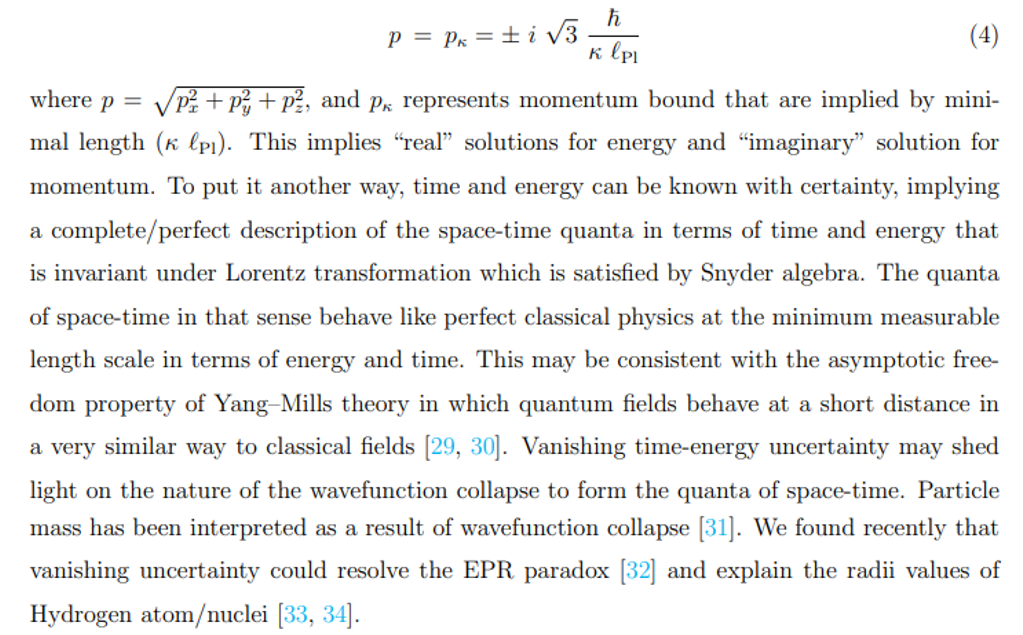
\ On another side, Bekenstein found a universal bound [35–37] that defines the maximal amount of information that is necessary to perfectly and completely describes a physical object up to the quantum level. Bekenstein universal bound is given by:
\
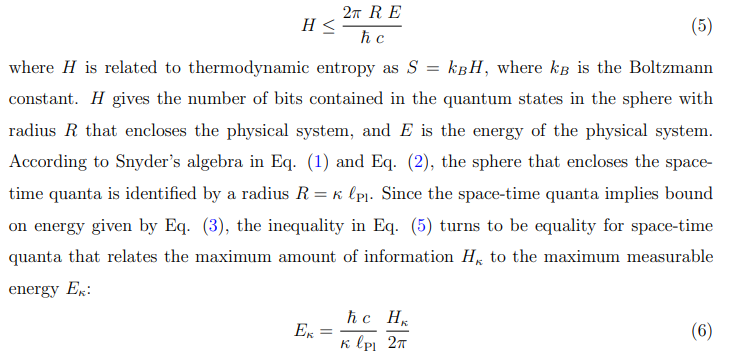
\ When we compare Eq. (3) with Eq. (6), we get:
\

\ that completely describes the quanta of space-time. Notice here that Hκ depends only on π and is independent of κ and nature constants.
\
:::info This paper is available on arxiv under CC BY 4.0 DEED license.
:::
\
This content originally appeared on HackerNoon and was authored by Phenomenology
Phenomenology | Sciencx (2024-07-31T15:10:51+00:00) Unpacking Space-Time Quanta: Snyder’s Algebra Meets Bekenstein’s Bound. Retrieved from https://www.scien.cx/2024/07/31/unpacking-space-time-quanta-snyders-algebra-meets-bekensteins-bound/
Please log in to upload a file.
There are no updates yet.
Click the Upload button above to add an update.
