This content originally appeared on HackerNoon and was authored by Extrapolate
:::info Author:
(1) K. Razi Naqvi, Department of Physics, Norwegian University of Science and Technology (NTNU), 7094 Trondheim, Norway
:::
Table of Links
3 Comments elicited by the solution to Milne’s problem for B-particles
6 Concluding remarks and References
2 Preliminary material
2.1 Statement of the Milne problem and terminology
A homogeneous, semi-infinite, non-absorbing medium occupies the half-space 𝑥 > 0, and sustains a constant current of test particles in the negative 𝑥 direction. The medium (or the host) itself contains no sources or sinks, and the plane boundary at 𝑥 = 0 acts as a black wall that absorbs all particles incident on it. The test particles obey either the one-speed LorentzBoltzmann equation (LBE) of neutron transport or radiative transfer, in which case one is faced with the Milne problem, or the Klein-Kramers equation (KKE), which leads one to the Brownian analog of the Milne problem. The problem is to determine 𝑛(𝑥), the density of the particles inside the medium (𝑥 > 0).
\ As two versions of the KKE have been brought to bear on the Milne problem, the label Klein-Kramers equation needs some elaboration. When Fermi analyzed Milne’s problem, in the context of neutron diffusion [9, pp. 980–1016], he decided to simplify the analysis “by considering the fictitious case in which the neutrons move in one dimension along a line, instead of being free in space.” The choice, he explained, “has the the advantage that we can easily obtain approximate expressions valid for the actual three-dimensional case.” The equation used by B&T applies to true one-dimensional Brownian motion. The Trondheim group (myself and two colleagues) have investigated this version [10] as well as the complete analog of the Milne problem, in which particles move in three-dimensional space, but plane symmetry prevails and the cocentration depends on only one cartesian coordinate [11]. Menon, Kumar and Sahni [12] pointed out that, as regards particle density, the two versions stand on an equal footing, but their difference does manifest itself when other physical moments are computed.
\
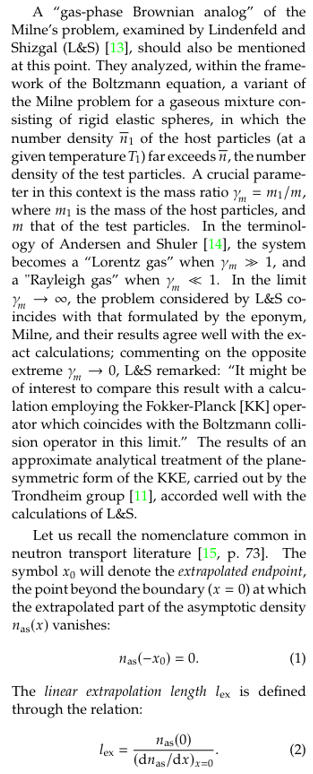
\ The models described by the KKE and the LBE lie at opposite poles. The latter has been called “inverse Brownian motion” [14, 16], and Hoare [17] refined the terminology by referrring to the former regular Brownian motion. A diffusing particle will be called a “B-particle” or an “L-particle” according as it obeys the KKE or LBE. Neither of these models is regarded as too remote to resemble a real physical system; the KKE is widely believed to provide a serviceable description of the thermal wanderings of a large particle (such as a colloid suspended in a liquid), and the LBE has found numerous applications in the transport of photons through a turbid medium or of a neutron through a moderator.
\
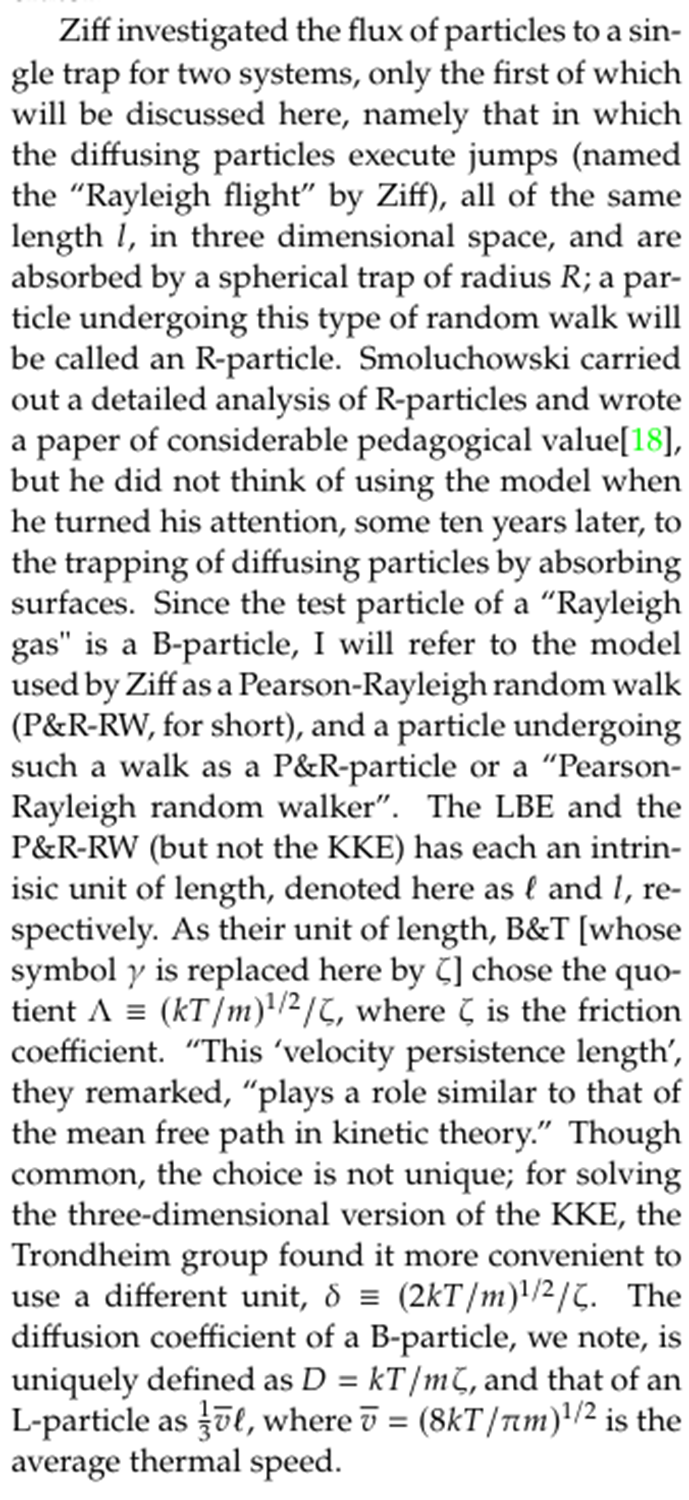
2.2 Results for the particle density
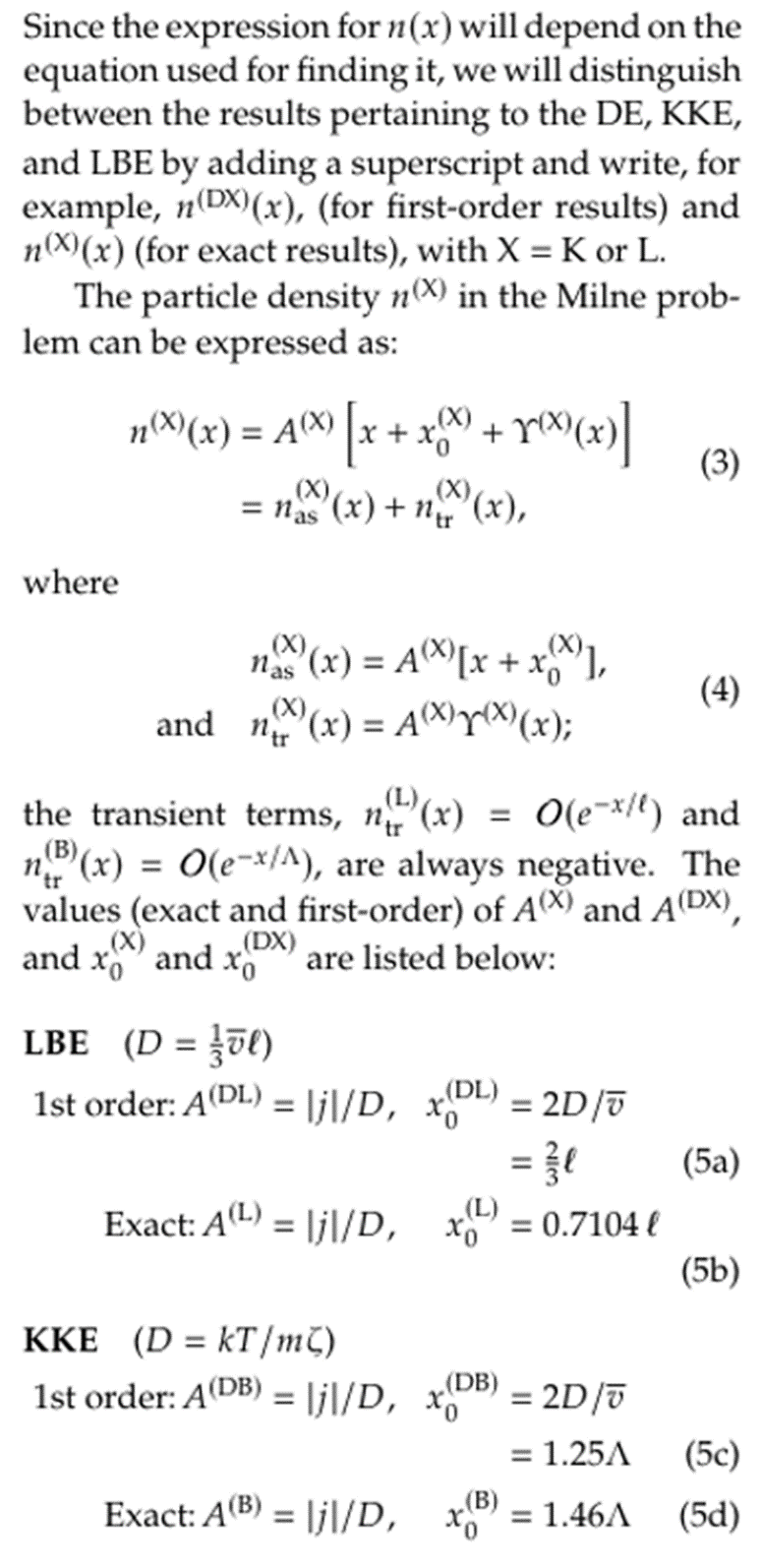
\
:::info This paper is available on arxiv under CC BY 4.0 DEED license.
:::
\
This content originally appeared on HackerNoon and was authored by Extrapolate
Extrapolate | Sciencx (2024-09-04T12:00:17+00:00) Revisiting Discrepancies in Diffusion Theory: Preliminary Material. Retrieved from https://www.scien.cx/2024/09/04/revisiting-discrepancies-in-diffusion-theory-preliminary-material/
Please log in to upload a file.
There are no updates yet.
Click the Upload button above to add an update.
