This content originally appeared on HackerNoon and was authored by Keynesian Technology
:::info Authors:
(1) Edward Crane, School of Mathematics, University of Bristol, BS8 1TH, UK;
(2) Stanislav Volkov, Centre for Mathematical Sciences, Lund University, Box 118 SE-22100, Lund, Sweden.
:::
Table of Links
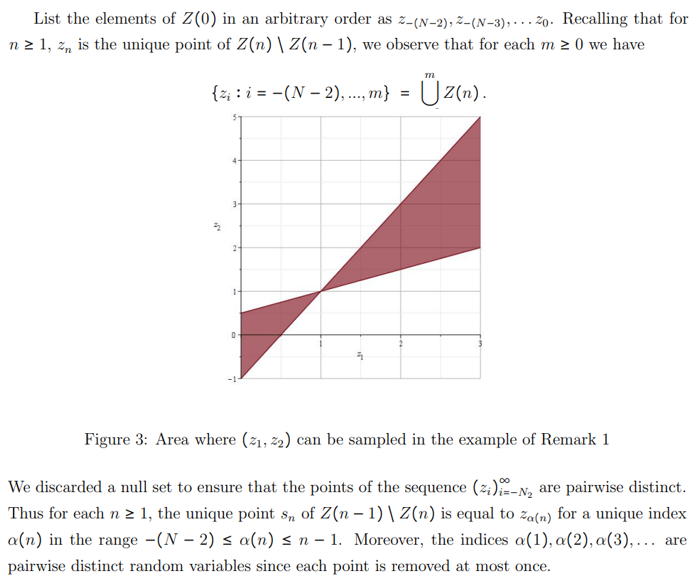
Reduction to the case of uniform geometry
All original points are eventually removed, a. s.
Acknowledgements and References
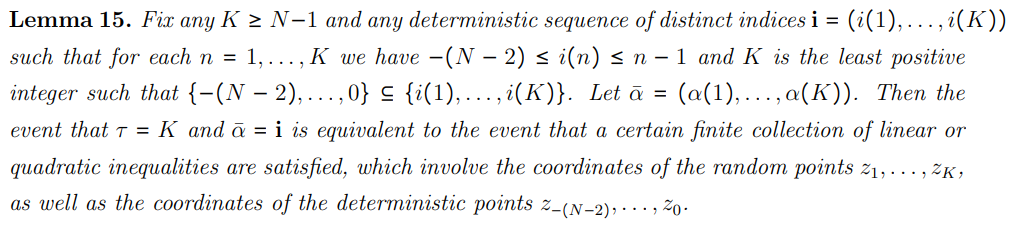
5 Proof of Theorem 1
Without loss of generality, we assume that the initial state Z(0) is deterministic. This is harmless since if for each deterministic choice of Z(0) the limit z∞ exists a.s. and has an absolutely continuous distribution, then if instead Z(0) is random, z∞ still exists a.s. and its distribution is a mixture of absolutely continuous distributions, which is necessarily absolutely continuous.
\


\
:::info This paper is available on arxiv under CC 4.0 license.
:::
\
7 i.e., a set defined by a number of polynomial inequalities and equalities; in our case, a.s. these will be just inequalities.
\ \
This content originally appeared on HackerNoon and was authored by Keynesian Technology
Keynesian Technology | Sciencx (2024-09-11T19:00:19+00:00) Analysis of the Jante’s Law Process and Proof of Conjecture: Proof of Theorem 1. Retrieved from https://www.scien.cx/2024/09/11/analysis-of-the-jantes-law-process-and-proof-of-conjecture-proof-of-theorem-1/
Please log in to upload a file.
There are no updates yet.
Click the Upload button above to add an update.
