This content originally appeared on HackerNoon and was authored by Computational Technology for All
Table of Links
4 Members of Deep Π0 1 classes
\

\
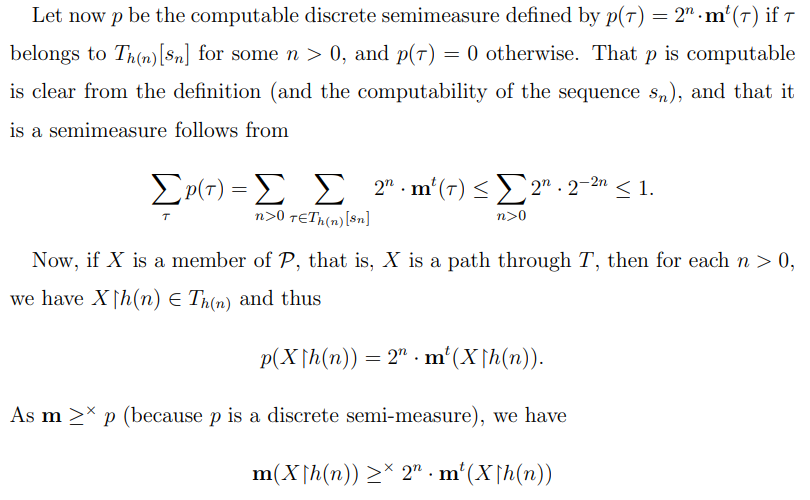
\ By Lemma 3, we can conclude that X is order-deep.
\ One immediate consequence of Theorem 9 is the following.
\

\ The converse of this result does not hold.
\
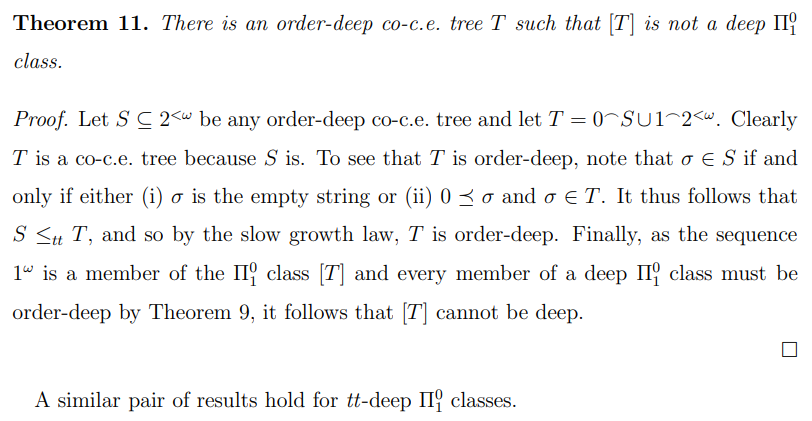
\
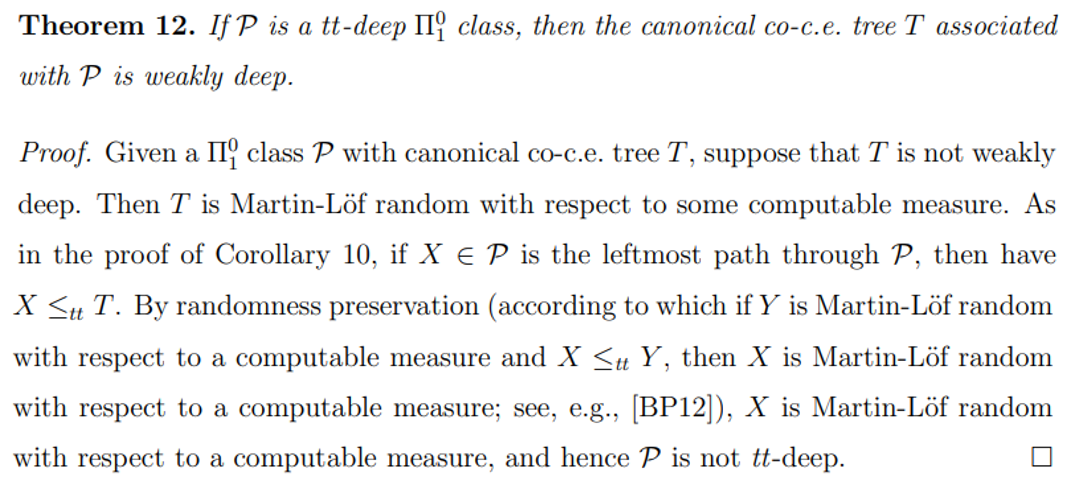
\
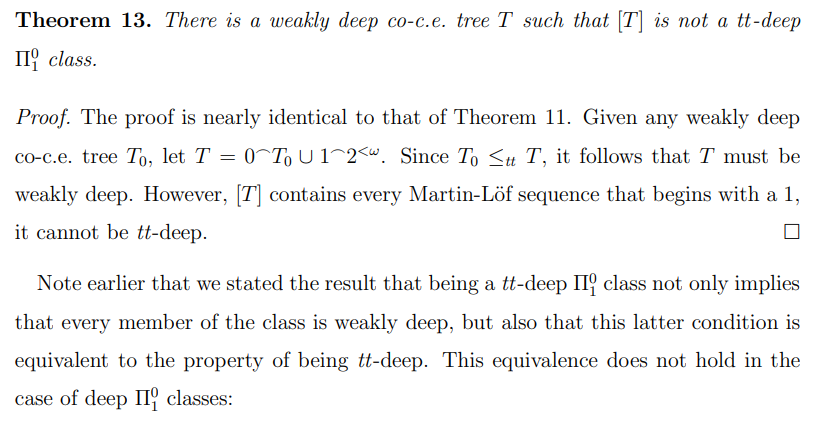
\

\ As an immediate consequence of Theorem 9 and the above results from [BP16], we have:
\
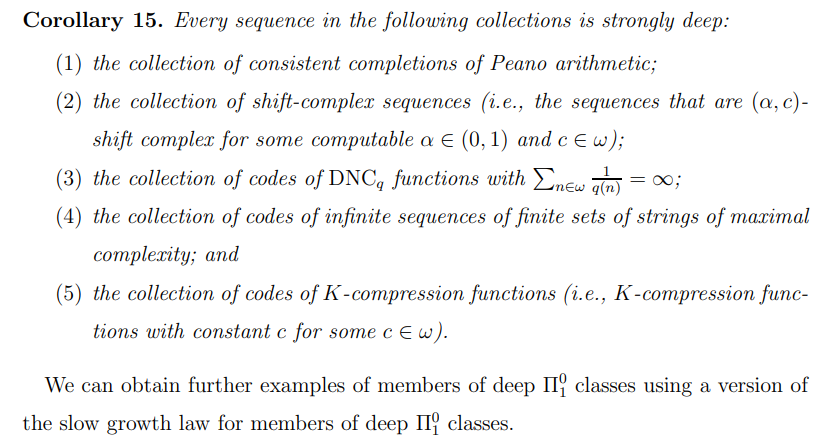
\

\
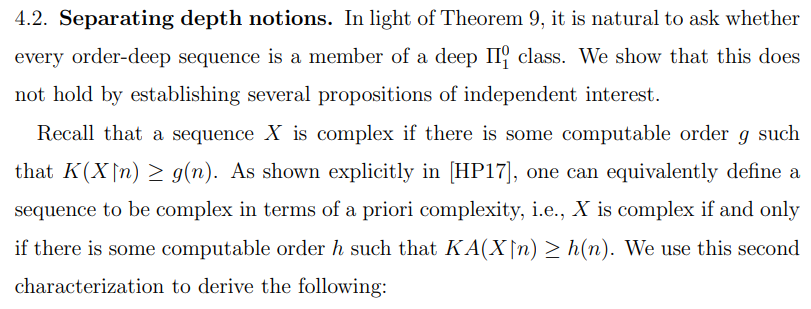
\
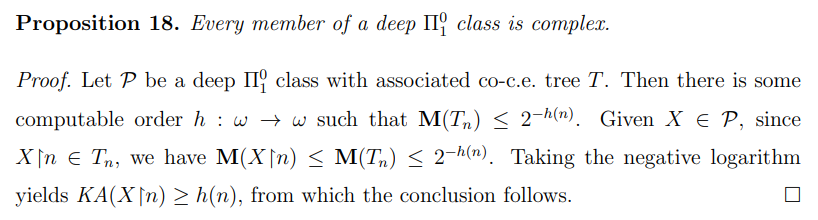
\ Next, we have:
\
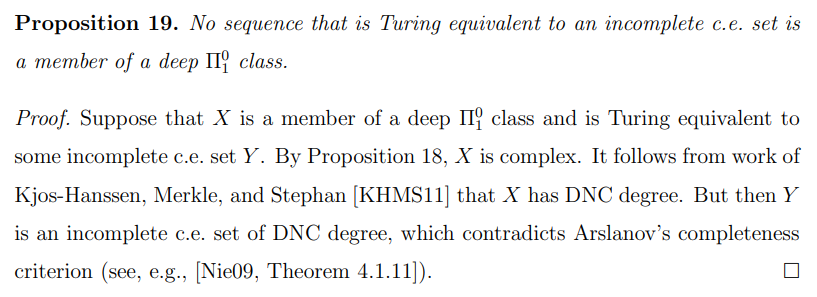
\
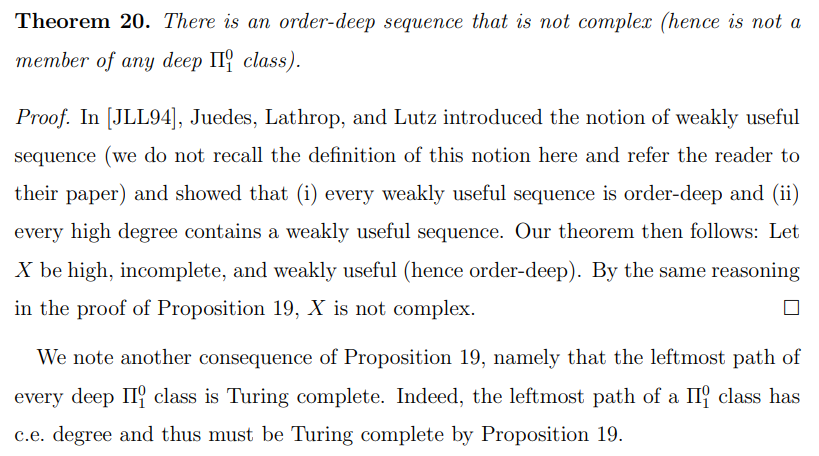
\

\

\
:::info This paper is available on arxiv under CC BY 4.0 DEED license.
:::
:::info Authors:
(1) Laurent Bienvenu;
(2) Christopher P. Porter.
:::
\
This content originally appeared on HackerNoon and was authored by Computational Technology for All
Computational Technology for All | Sciencx (2025-01-16T01:09:44+00:00) Bridging Computational Notions of Depth: Members of Deep Classes. Retrieved from https://www.scien.cx/2025/01/16/bridging-computational-notions-of-depth-members-of-deep-classes/
Please log in to upload a file.
There are no updates yet.
Click the Upload button above to add an update.
