This content originally appeared on HackerNoon and was authored by Linearization Technology
Table of Links
2. Mathematical Description and 2.1. Numerical Algorithms for Nonlinear Equations
2.4. Matrix Coloring & Sparse Automatic Differentiation
3.1. Composable Building Blocks
3.2. Smart PolyAlgortihm Defaults
3.3. Non-Allocating Static Algorithms inside GPU Kernels
3.4. Automatic Sparsity Exploitation
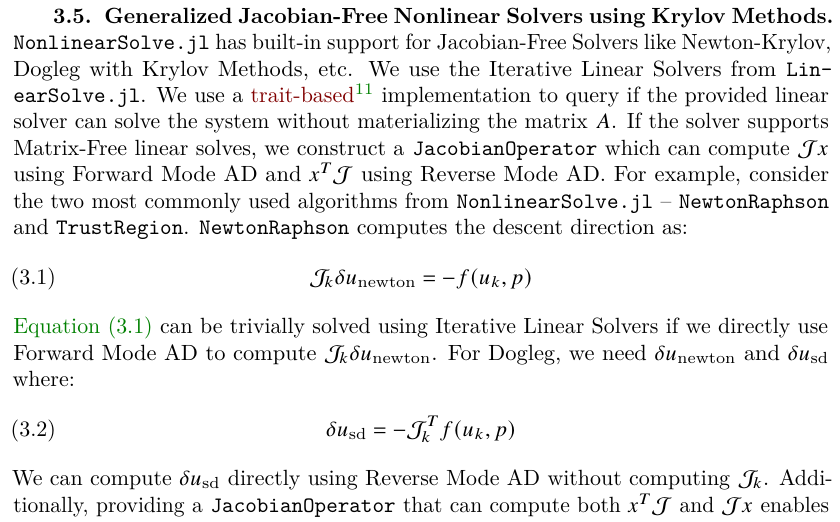
3.5. Generalized Jacobian-Free Nonlinear Solvers using Krylov Methods
4. Results and 4.1. Robustness on 23 Test Problems
4.2. Initializing the Doyle-Fuller-Newman (DFN) Battery Model
4.3. Large Ill-Conditioned Nonlinear Brusselator System
\
\
![Fig. 8: Work Precision Diagrams for 23 Test Problems: NonlinearSolve.jl Solvers solve all of the 23 test problems. Our solvers perform runtime checks against various system parameters [Subsection 3.2] and dynamically select internal solvers to outperform other solvers like Sundials, MINPACK, and NLsolve.jl.](https://cdn.hackernoon.com/images/fWZa4tUiBGemnqQfBGgCPf9594N2-sc93wa1.png)
\ us to use Least Squares Krylov Methods like LSMR efficiently. For certain Krylov Methods to converge, it is imperative to use Linear Preconditioning, which often requires a materialized Jacobian. In such cases, we provide an external control – concrete_jac – that overrides the default choice between materialized Jacobian and JacobianOperator and forces a concrete materialized Jacobian if set to true. In Subsection 4.3, we demonstrate the use of Jacobian-Free Newton and Dogleg Methods with GMRES [51] and preconditioning from IncompleteLU.jl and AlgebraicMultigrid.jl. We show that for large-scale systems, Krylov Methods [Figure 11] significantly outperform other methods [Figure 10]. Additionally, all our sparse Jacobian tooling is compatible with the Krylov Solvers, allowing us to generate cheaper sparse Jacobians for the preconditioning.
\
:::info This paper is available on arxiv under CC BY 4.0 DEED license.
:::
[11] https://invenia.github.io/blog/2019/11/06/julialang-features-part-2/
:::info Authors:
(1) AVIK PAL, CSAIL MIT, Cambridge, MA;
(2) FLEMMING HOLTORF;
(3) AXEL LARSSON;
(4) TORKEL LOMAN;
(5) UTKARSH;
(6) FRANK SCHÄFER;
(7) QINGYU QU;
(8) ALAN EDELMAN;
(9) CHRIS RACKAUCKAS, CSAIL MIT, Cambridge, MA.
:::
\
This content originally appeared on HackerNoon and was authored by Linearization Technology
Linearization Technology | Sciencx (2025-03-28T01:20:16+00:00) Generalized Jacobian-Free Nonlinear Solvers Using Krylov Methods. Retrieved from https://www.scien.cx/2025/03/28/generalized-jacobian-free-nonlinear-solvers-using-krylov-methods/
Please log in to upload a file.
There are no updates yet.
Click the Upload button above to add an update.
