This content originally appeared on HackerNoon and was authored by Gamifications
:::info Authors:
(1) Chengfeng Shen, School of Mathematical Sciences, Peking University, Beijing;
(2) Yifan Luo, School of Mathematical Sciences, Peking University, Beijing;
(3) Zhennan Zhou, Beijing International Center for Mathematical Research, Peking University.
:::
Table of Links
2 Model and 2.1 Optimal Stopping and Obstacle Problem
2.2 Mean Field Games with Optimal Stopping
2.3 Pure Strategy Equilibrium for OSMFG
2.4 Mixed Strategy Equilibrium for OSMFG
3 Algorithm Construction and 3.1 Fictitious Play
3.2 Convergence of Fictitious Play to Mixed Strategy Equilibrium
3.3 Algorithm Based on Fictitious Play
4 Numerical Experiments and 4.1 A Non-local OSMFG Example
5 Conclusion, Acknowledgement, and References
2.2 Mean Field Games with Optimal Stopping
Mean field games (MFG) are a class of mathematical models that study strategic decision-making by a large number of agents, where each agent’s decision is affected by the average behavior of all the other agents. Here, the collection of agents is also referred to as the crowd. Mean field games with optimal stopping (OSMFG) are a type of mean field game where the agents in the game must choose when to stop playing, in order to minimize their expected cost. What we are interested in is the equilibrium of OSMFGs.
\ Generally speaking, one constructs an equilibrium by three steps in MFGs. First, all agents guess how the distribution of the crowd would propagate in [0, T ]. Second, all agents take the optimal control respectively based on the guessed propagation. This would induce the actual propagation of the distribution of the crowd. Third, we check whether the actual propagation coincides with the guessed one. If they are the same, we say the system attains an equilibrium.
\
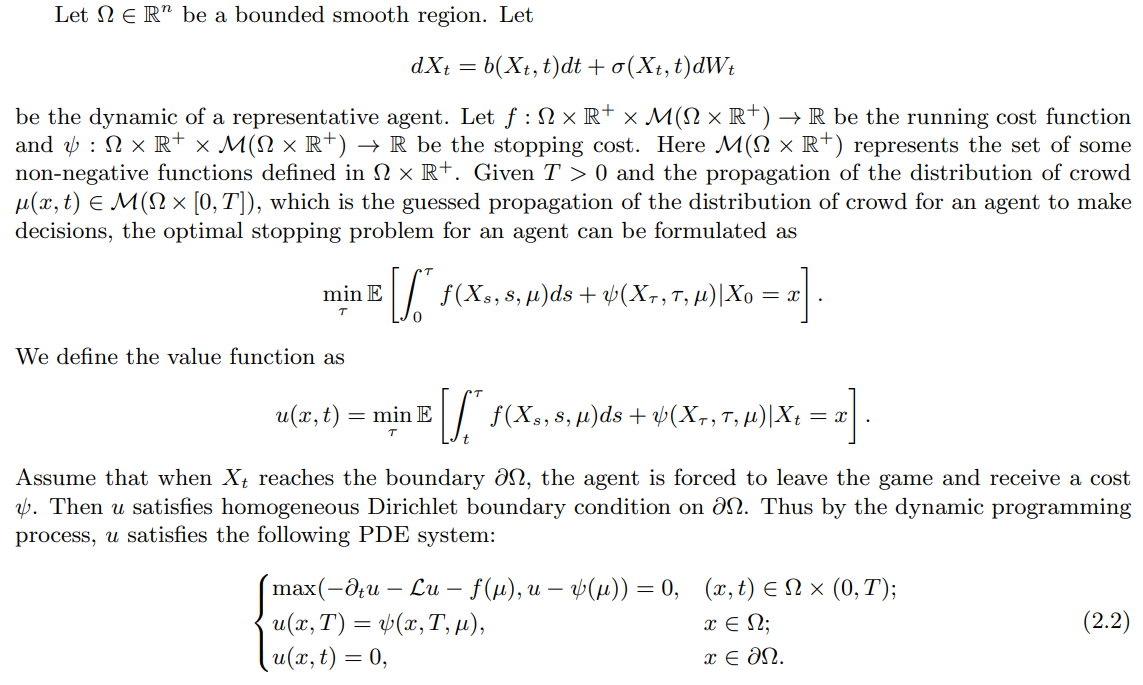
\ The optimal control would be implied from (2.2). On the other hand, given the optimal control taken by all agents, we can solve a Fokker-Planck equation to obtain the actual distribution of crowd m. When m = µ, we say the system attains an equilibrium.
\ It is worth emphasizing that the optimal control might not be unique. Agents may have multiple optimal strategies, which can lead to multiple actual propagations of the crowd and hence multiple notions of equilibrium. We would introduce the pure strategy equilibrium and the mixed strategy equilibrium in the next two parts.
\
:::info This paper is available on arxiv under CC 4.0 license.
:::
\
This content originally appeared on HackerNoon and was authored by Gamifications
Gamifications | Sciencx (2024-09-25T13:00:27+00:00) Fictitious Play for Mixed Strategy Equilibria in Mean Field Games: Mean Field Games. Retrieved from https://www.scien.cx/2024/09/25/fictitious-play-for-mixed-strategy-equilibria-in-mean-field-games-mean-field-games/
Please log in to upload a file.
There are no updates yet.
Click the Upload button above to add an update.
